Voltage Drop Calculation for Single Phase and Three Phase Systems
 |
| Electric Panel Board |
When it comes to voltage drop calculations, there are several valuable individual approach that can be used when working with electrical systems. Thus, it is important for an engineer to determine to match his calculations against the actual scenario in the type system or type of installation. The following scenario covers comprehensive calculation involving electric circuits in different scenario.
Example 1. Calculate voltage drop in a single-phase dc circuit.
A solid #14 copper two-wire cable that is 750 feet long supplies a 125 ohm load resistor for heating purposes at 75 deg. C. Find the voltage drop in the cable; and find the resulting voltage across the load resistor.
 |
| Diagram: DC Circuit |
Solution:
Calculate the cable resistance, for the sake of illustration, the overall length need to be multiplied by two to consider the cable resistance (out) and cable resistance (back). Assume that the cable has a uniform resistance of 75 Deg. C.
Solve for cable resistance,
Cable resistance = (RESISTANCE FOR 1000 FT.) x (750 / 1000) x 2
- R = (3.07 ohms per M ft.) X (0.750) x 2
- R = 2.3 ohms x 2
- R = 4.6 ohms.
Total resistance = 125ohms + 2.3 ohms = 129.6 ohms
Current = 125/ 129.6 = 0.9645 Amperes
Voltage drop in cable = current x cable resistance
- Vd = (0.9645) X (4.6)
Therefore,
- Voltage Drop in the Cable = 4.436 V
- Voltage at the load terminal = 0.9645 x 125 ohms = 120.56 Volts
 |
| Diagram: AC circuit |
Cable resistance = (RESISTANCE FOR 1000 FT.) x (750 / 1000) x 2
- R = (1.70 ohms per M ft.) X (0.750) x 2
- R = 1.275 ohms x 2
- R = 2.55 ohms.
Calculate the total current flowing in the circuit.
- I = 1,300/ 115 (ignoring power factor)
- I = 11.3 Ampere
Voltage drop in cable = current x cable resistance
- Vd = (11.3) X (2.55 ohms)
- Vd = 28.82 Volts
Therefore,
- Voltage Drop in the Cable = 28.82 Volts
- Voltage at the load terminal = 115 - 28.82 = 86.18 Volts
 |
| Diagram: 3- phase AC circuit |
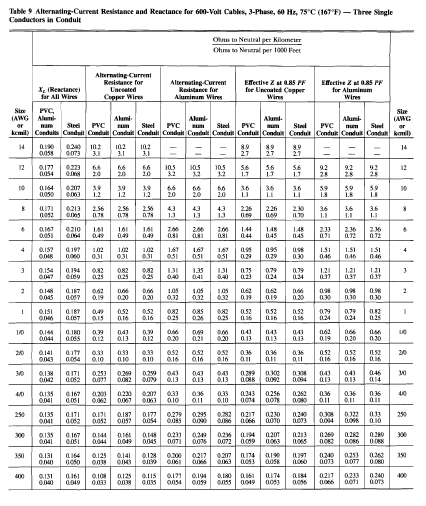
Cable resistance = (RESISTANCE FOR 1000 FT.) x (280 / 1000) - per line
- R = (0.078 ohms per M ft.) X (0.0.28)
- R = 0.0218 ohms - per line
The current is given at 200 Amperes.
Voltage drop in cable = current x cable resistance
- Vd = (200) X (0.0218 ohms) x 1.73 (multiple a factor 1.73 for 3 phase systems)
- Vd = 7.55 Volts
Therefore,
- Voltage Drop in the Cable = 7.55 Volts
- Voltage at the motor terminals = 480 - 7.55 = 472.45 Volts


.webp)










No comments: